 |
Professor Nonlinear Physical Chemistry Unit Service de Chimie Physique et Biologie Théorique, and Center for Nonlinear Phenomena and Complex Systems (CENOLI) Faculté des Sciences, Campus Plaine, C.P. 231 Université libre de Bruxelles, 1050 Brussels, Belgium ☎ +32 (0)2 650 5784 📠 +32 (0)2 650 5767 ✉ yannick.de.decker@ulb.be |
It is now well known that reactive
systems maintained far from thermodynamic equilibrium can give rise
to complex organized behaviors. Such organizations include regular
oscillations of concentrations in time and/or space, explosive
phenomena, complex chemical kinetics, etc.
From a theoretical point of view, these systems can be modeled with the laws governing the macroscopic behavior of matter, which typically take the form of reaction-diffusion-advection equations. This level of description is appropriate and satisfactory for most of the cases where the observed organization involves macroscopic length scales (millimeters to meters) and time scales (miilisecond to hours or days). But it leaves many questions unanswered. What are the intrinsic limitations of the laws that are used? Where do they come from? Can they still be used for much smaller and faster systems, or does one need to amend them in such cases? Can organization exist at all in micrometric or nanometric reactive systems? Answering these questions is important not only for a better fundamental understanding of chemical dynamics, but it is also pivotal to the development of nanotechnologies and the modeling of biological cells.
In this context, my main research objective is to arrive to a coherent and multiscale understanding of the dynamical behavior of non-equilibrium reactive systems. To connect the different scales with each other, I rely on a stochastic description of the dynamics, in which all the processes are seen as random events characterized by well-defined probabilities. This leads to a new theoretical framework that allows one to extend thermodynamics and kinetics to the nanoscale. This approach has undergone tremendous progress in the last decades, thanks to the work of many research groups all over the world. My contribution to this effort focuses mainly (and up to this day) on three important aspects, as described below.
From a theoretical point of view, these systems can be modeled with the laws governing the macroscopic behavior of matter, which typically take the form of reaction-diffusion-advection equations. This level of description is appropriate and satisfactory for most of the cases where the observed organization involves macroscopic length scales (millimeters to meters) and time scales (miilisecond to hours or days). But it leaves many questions unanswered. What are the intrinsic limitations of the laws that are used? Where do they come from? Can they still be used for much smaller and faster systems, or does one need to amend them in such cases? Can organization exist at all in micrometric or nanometric reactive systems? Answering these questions is important not only for a better fundamental understanding of chemical dynamics, but it is also pivotal to the development of nanotechnologies and the modeling of biological cells.
In this context, my main research objective is to arrive to a coherent and multiscale understanding of the dynamical behavior of non-equilibrium reactive systems. To connect the different scales with each other, I rely on a stochastic description of the dynamics, in which all the processes are seen as random events characterized by well-defined probabilities. This leads to a new theoretical framework that allows one to extend thermodynamics and kinetics to the nanoscale. This approach has undergone tremendous progress in the last decades, thanks to the work of many research groups all over the world. My contribution to this effort focuses mainly (and up to this day) on three important aspects, as described below.
The chemical kinetics of spatially constrained systems
The origin of the macroscopic laws
is well understood in the case of relatively dilute reactive
systems, including the case of aqueous solutions of reactants.
However, it has been observed through simulations and experiments
that the usual reaction-diffusion-convection equations do not work
properly when particles are placed in a spatially constrained
environment. The most striking example of this is probably the case
of reactions taking place on a surface, a situation encountered
among others in heterogeneous catalysis.
It is however possible to derive amended evolution laws that offer a much better agreement with simulations and experiments. We used the a lattice-compatible stochastic description to derive explicitly such corrections in the case of simple systems [1-5], and to model more complex systems such as the explosive behavior observed in microscopy experiments, in collaboration with the group of N. Kruse (ULB) [6].
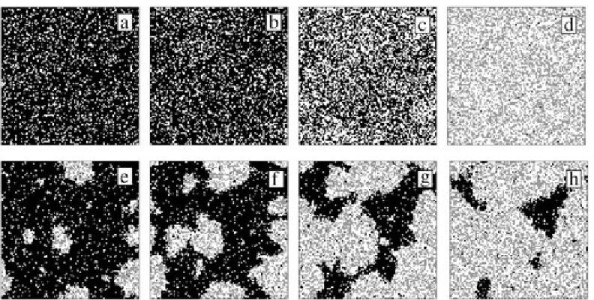
Figure 1: Kinetic Monte Carlo simulation of an explosive transition from a NO-covered Pt surface to an almost empty surface
(NO molecules appear black, H adatoms appear grey and empty adsorption sites are blank)
The corrections obtained in this way highlight the central role played by spontaneous fluctuations of composition, which alter the usual macroscopic picture: Local variations of the composition can build up instead of being washed away, because the transport of particles is inefficient in restricted geometries. In spite of their success, evolution laws obtained in this way are not easy to apply because of their intrinsic complexity: They are based on an infinite hierarchy of evolution equations for the statistical quantities of interest. There exists as of today no general and systematic way to close such hierarchy with a controlled error on the final result. We are now investigating how such systematic procedures could be built by relying, for example, on the existence of low-dimensional inertial manifolds in the a priori infinite dimensional phase space.
The robustness of chemical nanodynamics
Regular temporal oscillations of a system's composition have been reported repeatedly for non-equilibrium reactions. They have been observed in systems as small as single biological cells or in atomic microscopy experiments. This last feature is to some extent unexpected. In small systems, the low number of particles makes the concentrations "jump" by quite large finite steps, leading to an intrinsically noisy dynamics. So, one could wonder why nanometric chemical clocks are so robust.
Theoretical predictions can be made, based on the available literature, on how fluctuations affect the regularity of the period and of the amplitude of chemical oscillators. Our objective here consists in assessing the validity of such theoretical predictions by performing extensive analyses and stochastic modeling of experimental data obtained by real-time atomic microscopy. Using the time series of an experimental signal, we could for example reconstruct the phase space trajectories and the attractor of a chemical nanoclock (the NO2+H2 reaction on platinum [7,8]).
It is however possible to derive amended evolution laws that offer a much better agreement with simulations and experiments. We used the a lattice-compatible stochastic description to derive explicitly such corrections in the case of simple systems [1-5], and to model more complex systems such as the explosive behavior observed in microscopy experiments, in collaboration with the group of N. Kruse (ULB) [6].

Figure 1: Kinetic Monte Carlo simulation of an explosive transition from a NO-covered Pt surface to an almost empty surface
(NO molecules appear black, H adatoms appear grey and empty adsorption sites are blank)
The corrections obtained in this way highlight the central role played by spontaneous fluctuations of composition, which alter the usual macroscopic picture: Local variations of the composition can build up instead of being washed away, because the transport of particles is inefficient in restricted geometries. In spite of their success, evolution laws obtained in this way are not easy to apply because of their intrinsic complexity: They are based on an infinite hierarchy of evolution equations for the statistical quantities of interest. There exists as of today no general and systematic way to close such hierarchy with a controlled error on the final result. We are now investigating how such systematic procedures could be built by relying, for example, on the existence of low-dimensional inertial manifolds in the a priori infinite dimensional phase space.
The robustness of chemical nanodynamics
Regular temporal oscillations of a system's composition have been reported repeatedly for non-equilibrium reactions. They have been observed in systems as small as single biological cells or in atomic microscopy experiments. This last feature is to some extent unexpected. In small systems, the low number of particles makes the concentrations "jump" by quite large finite steps, leading to an intrinsically noisy dynamics. So, one could wonder why nanometric chemical clocks are so robust.
Theoretical predictions can be made, based on the available literature, on how fluctuations affect the regularity of the period and of the amplitude of chemical oscillators. Our objective here consists in assessing the validity of such theoretical predictions by performing extensive analyses and stochastic modeling of experimental data obtained by real-time atomic microscopy. Using the time series of an experimental signal, we could for example reconstruct the phase space trajectories and the attractor of a chemical nanoclock (the NO2+H2 reaction on platinum [7,8]).
 |
 |
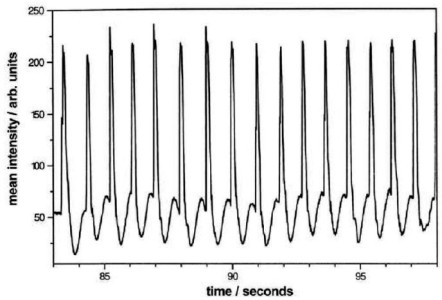
Figure
2: Left, from [7]: Temporal evolution of the
brightness on a (210) facet during self-sustained oscillations
in the NO2(g)+H2(g)/Pt reaction at 400K. Right, from [8]:
"Stochastic volcano" showing the distribution of fluctuations
around the underlying limit cycle in a reconstructed phase
space (the vertical axis is the probability of occurrence and
the horizontal coordinates are delayed signals).
We could prove in this way the existence of a so-called "noisy" limit cycle, whose existence was previously predicted by stochastic approaches. We could also verify a scaling law, proposed by P. Gaspard, which connects the variance of the period to the half-time of the autocorrelation function of the signal.
This reactive system is especially rich and needs further investigations. In the experiments, the oscillations are seen to emerge suddenly after a critical combination of parameters is satisfied. A detailed mechanistic model will be necessary in order to understand the origin of such a bifurcation. Such a model should also be able to explain why the amplitude of fluctuations around the cycle changes as a function of the advancement of the reaction, as can be seen in Figure 2.
The collective dynamics of nano-assemblies
In the now famous talk he gave at CalTech in 1959, Richard Feynman envisioned the consequences that an atomic level control of matter could have on technology. The world had to wait until the eighties, though, before significant steps towards such nanotechnologies could be made. As of today, these new technologies have invaded many aspects of our life and are used in fields as varied as electronics, pharmacy, or materials science.
Major progress could recently be achieved by relying not only on the structural characteristics but also on the dynamical properties of supramolecular assemblies. New physicochemical features could be observed for those nanoparticles that respond dynamically to external stimuli. Nanostructured materials with switchable functions have for example been synthesized, with potential applications for catalysis or electronics. In order for this emerging field to fully develop, it will be mandatory to endow it with a rigorous theoretical framework, the focus of which should be on the dynamical properties of the system.
This project aims at developing the theoretical basis of such non-equilibrium thermodynamics and kinetics of reactive nano-objects. It is, as of today, still a work in progress. Our objective is to first investigate the combination of self-assembly and self-organization in the case of some simple, toy models [9-12].
We could prove in this way the existence of a so-called "noisy" limit cycle, whose existence was previously predicted by stochastic approaches. We could also verify a scaling law, proposed by P. Gaspard, which connects the variance of the period to the half-time of the autocorrelation function of the signal.
This reactive system is especially rich and needs further investigations. In the experiments, the oscillations are seen to emerge suddenly after a critical combination of parameters is satisfied. A detailed mechanistic model will be necessary in order to understand the origin of such a bifurcation. Such a model should also be able to explain why the amplitude of fluctuations around the cycle changes as a function of the advancement of the reaction, as can be seen in Figure 2.
The collective dynamics of nano-assemblies
In the now famous talk he gave at CalTech in 1959, Richard Feynman envisioned the consequences that an atomic level control of matter could have on technology. The world had to wait until the eighties, though, before significant steps towards such nanotechnologies could be made. As of today, these new technologies have invaded many aspects of our life and are used in fields as varied as electronics, pharmacy, or materials science.
Major progress could recently be achieved by relying not only on the structural characteristics but also on the dynamical properties of supramolecular assemblies. New physicochemical features could be observed for those nanoparticles that respond dynamically to external stimuli. Nanostructured materials with switchable functions have for example been synthesized, with potential applications for catalysis or electronics. In order for this emerging field to fully develop, it will be mandatory to endow it with a rigorous theoretical framework, the focus of which should be on the dynamical properties of the system.
This project aims at developing the theoretical basis of such non-equilibrium thermodynamics and kinetics of reactive nano-objects. It is, as of today, still a work in progress. Our objective is to first investigate the combination of self-assembly and self-organization in the case of some simple, toy models [9-12].
 |
 |
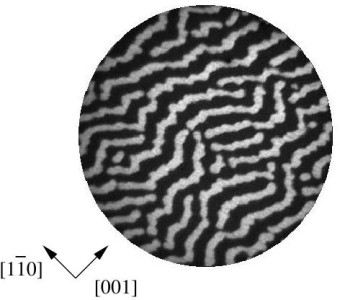
Figure
3: Left: PEEM image of a stationary potassium
concentration pattern observed during the H2+O2
reaction
on a K-covered Rh surface at 565 K. The diameter of the imaged area is 400 micrometers.
Right: Potassium distribution obtained with a simple reaction-diffusion model taking lateral interactions into account (from [9]).
on a K-covered Rh surface at 565 K. The diameter of the imaged area is 400 micrometers.
Right: Potassium distribution obtained with a simple reaction-diffusion model taking lateral interactions into account (from [9]).
We will then try to
prove that a detailed analysis of the collective
dynamics of nanoparticles can be performed routinely for
more complex systems, by relying directly and only
on microscopic simulations [13]. The multiscale
numerical approach at the heart of this project will be
applied on the case of a surface nanostructuring process
whose chemistry and physics are well characterized,
namely the reactive control of self-assembled vanadium
oxides on rhodium.
- Y. De Decker, G. A. Tsekouras, Th. Erneux, A. Provata and G. Nicolis, Phys. Rev. E 69, 036203 (2004) and Vir. J. Bio. Phys. Res. 7 (7) (2004).
- Y. De Decker and F. Baras, EPJ B 78, 173 (2010).
- X. Guo, Y. De Decker and J.W. Evans, Phys. Rev. E 82, 021121 (2010).
- D. Bullara, Y. De Decker and R. Lefever, Phys. Rev. E 87, 062923 (2013).
- D. Bullara and Y. De Decker, How does the microscopic mechanism of nonlinear reactions affects the range of validity of the mass action law? in preparation.
- Y. De Decker, F. Baras, N. Kruse and G. Nicolis, J. Chem. Phys. 117, 10244 (2002).
- J.-S. McEwen, P. Gaspard, Y. De Decker, C. Barroo, T. Visart de Bocarmé and N. Kruse, Langmuir 26, 16381 (2010).
- C. Barroo, T. Visart de Bocarmé, P. Gaspard, N. Kruse, and Y. De Decker, Robust chemical oscillations at the nanoscale, in preparation.
- Y. De Decker, H. Marbach, M. Hinz, S. Günther, M. Kiskinova, A.S. Mikhailov and R. Imbihl, Phys. Rev. Lett. 92, 198305 (2004).
- Y. De Decker and A.S. Mikhailov, J. Phys. Chem. B 108, 14759 (2004).
- Y. De Decker and A.S. Mikhailov, Prog. Theoret. Phys. – Supp. 165, 119 (2006).
- I. Berenstein and Y. De Decker, J. Chem. Phys. 143, 064105 (2015).
- G. Mazzi, Y. De Decker, and G. Samaey, J. Chem. Phys. 137, 204115 (2012).
- C. Robert, F. Prista von Bonhorst, Y. De Decker, G. Dupont, D.
Gonze
Initial source of heterogeneity in a model for cell fate decision in the early mammalian embryo, Interface Focus 12, 20220010 (2022). - J. Gillet, L. Rongy, Y. De Decker
Spontaneous mirror symmetry breaking in reaction–diffusion systems: ambivalent role of the achiral precursor, Phys. Chem. Chem. Phys. 24, 26144 (2022). - Y. De Decker, G. Nicolis
On the Fokker–Planck approach to the stochastic thermodynamics of reactive systems, Physica A 553, 124269 (2020). - A. Grau Ribes, Y. De Decker, L. Rongy
Connecting gene expression to cellular movement: A transport model for cell migration, Phys. Rev. E 100, 032412 (2019). - Y. De Decker
Stochastic thermodynamics based on an Einstein-Boltzmann definition of fluctuating entropy, Phys. Rev. E 99, 032143 (2019). - Y. De Decker, A. Raghamy, R. Imbihl
Modeling the Formation and Propagation of VOx Islands on Rh(111) under Reactive Conditions, J. Phys. Chem. C 123(18), 11602 (2019). - J.-F. Derivaux, Y. De Decker
Stochastic efficiency of thermodiffusion: an extended local equilibrium approach, J. Stat. Mech. Theory Exp. 034002 (2019). - D. Bullara, Y. De Decker, I. R. Epstein
On the possibility of spontaneous chemomechanical oscillations in adsorptive porous media, Phil. Trans. R. Soc. A 376, 20170374 (2018). - C. Barroo, V. Voorsluijs, T. Visart de Bocarmé, P. Gaspard, Y.
De Decker
Reconstructing stochastic attractors from nanoscale experiments on a non-equilibrium reaction, Phys. Chem. Chem. Phys. 20, 21302 (2018). - A. Grau Ribes, Y. De Decker, C. Gérard and L. Rongy
Modelling the propagation of a dynamical signature in gene expression mediated by the transport of extracellular microRNAs, Mol. BioSyst. 13, 2379 (2017). - V. Voorsluijs, I. G. Kevrekidis, and Y. De Decker
Nonlinear behavior and fluctuation-induced dynamics in the photosensitive Belousov-Zhabotinsky reaction, Phys. Chem. Chem. Phys. 19, 22528 (2017). - C. Barroo, Y. De Decker, L. Jacobs, and T. Visart de Bocarmé
Nonlinear behavior during NO2 hydrogenation on a nanosized Pt-Rh catalyst sample, Appl. Surf. Sci. 412, 546 (2017). - C. Barroo, Y. De Decker, and T. Visart de Bocarmé
NO2 Hydrogenation on Rh Catalysts: Bifurcations and Oscillations at the Nanoscale, J. Phys. Chem. C 121, 17235 (2017). - G. Nicolis and Y. De Decker
Stochastic thermodynamics of Brownian motion, Entropy 19, 434 (2017). - G. Nicolis and Y. De Decker
Stochastic approach to irreversible thermodynamics, Chaos 27, 104615 (2017). - C Barroo, T. Visart de Bocarmé, Y De Decker and N. Kruse
Surface Reactions Investigated at the Nanoscale by Field Emission Techniques: Nonlinear Dynamics of the Catalytic Hydrogenation of NO and NO2 Over Platinum Crystallites, Reference Module in Chemistry, Molecular Sciences and Chemical Engineering, 2017. - C. Barroo, L. Jacobs, N. Gilis, S. V. Lambeets, S. Owczarek, Y.
De Decker and T. Visart de Bocarmé
Field Emission Microscopy to Study the Catalytic Reactivity of Binary Alloys at the Nanoscale, Microsc. Microanal. 23, 610 (2017). - V. Voorsluijs and Y. De Decker
Emergence of chaos in a spatially confined reactive system, Physica D 335, 1 (2016). - Y. De Decker, J.-F. Derivaux and G. Nicolis
Stochastic thermodynamics of reactive systems: An extended local equilibrium approach, Phys. Rev. E 93, 042127 (2016). - I. Berenstein, C. Beta and Y. De Decker
Comment on "Flow-induced arrest of spatiotemporal chaos and transition to a stationary pattern in the Gray-Scott model", Phys. Rev. E 94, 046201 (2016). - C. Barroo, Y. De Decker, T. Visart de Bocarmé and N. Kruse
Emergence of Chemical Oscillations from Nanosized Target Patterns, Phys. Rev. Lett. 117, 144501 (2016). - S.R. de Paulo, I.J.C. de Paulo and Y. de Decker
Reconstructing the micrometeorological dynamics of the southern Amazonian transitional forest, Chaos 25, 123123 (2015). - D. Bullara and Y. De Decker
Chemical Equilibrium on Low Dimensional Supports: Connecting the Microscopic Mechanism to the Macroscopic Observations, J. Stat. Phys. 161, 210 (2015). - Y. De Decker, A.G. Cantù Ros and G. Nicolis
Extended local equilibrium approach to stochastic thermodynamics, EPJ ST 224, 947 (2015). - C. Barroo, Y. De Decker, T. Visart De Bocarmé and P. Gaspard
Fluctuating dynamics of nanoscale chemical oscillations: Theory and experiments, J. Phys. Chem. Lett. 6, 2189 (2015). - Y. De Decker
On the stochastic thermodynamics of reactive systems, Physica A 428, 178 (2015). - D. Bullara and Y. De Decker
Pigment cell movement is not required for generation of Turing patterns in zebrafish skin, Nature Communications 6, 6971 (2015). - I. Berenstein and Y. De Decker
Spatiotemporal chaos from bursting dynamics, J. Chem. Phys. 143, 064105 (2015). - Y. de Decker, D. Bullara, C. Barroo and T. Visart de Bocarmé
Nonlinear dynamics of reactive nanosystems: Theory and experiments, Springer Series in Materials Science 217, 127 (2015). - C. Barroo, Y. De Decker, T. Visart de Bocarmé and N. Kruse
NO2 Hydrogenation over Pt and Rh Catalysts: a Study at The Atomic Level by Field Emission Microscopy, Microsc. Microanal. 21, 1587 (2015). - I. Berenstein and Y. De Decker
Defect-mediated turbulence and transition to spatiotemporal intermittency in the Gray-Scott model, Chaos 24, 043109 (2014). - C. Barroo, Y. De Decker, T. Visart De Bocarmé and N. Kruse
Complex oscillation patterns during the catalytic hydrogenation of NO2 over platinum nanosized crystals, J. Phys. Chem. C 118, 6839 (2014). - I. Berenstein, D. Bullara and Y. De Decker
Stationary spots and stationary arcs induced by advection in a one-activator, two-inhibitor reactive system, Chaos 24 , 1 (2014). - C. Barroo, S.V. Lambeets, F. Devred, T.D. Chau, N. Kruse, Y. De
Decker and T. Visart De Bocarmé
Hydrogenation of NO and NO2 over palladium and platinum nanocrystallites: Case studies using field emission techniques, New J. Chem. 38, 2090 (2014). - C.I. Siettos, Y. De Decker and G.C. Georgiou
Complex systems modelling, analysis, and control, J. Appl. Math. 2013, 508176 (2013). - D. Bullara, Y. De Decker and R. Lefever
Nonequilibrium chemistry in confined environments: A lattice Brusselator model, Phys. Rev. E 87, 062923 (2013). - G. Mazzi, Y. De Decker and G. Samaey
Towards an efficient multiscale modeling of low-dimensional reactive systems: Study of numerical closure procedures, J. Chem. Phys. 137, 204115 (2012). - A.I. Reppas, Y. De Decker and C.I. Siettos
On the efficiency of the equation-free closure of statistical moments: Dynamical properties of a stochastic epidemic model on Erdos-Rényi networks, J. Stat. Mech. Theo. Exp. 2012, P08020 (2012). - C. Almarcha, Y. R'Honi, Y. De Decker, P.M.J. Trevelyan, K.
Eckert and A. De Wit
Convective mixing induced by acid-base reactions, J. Phys. Chem. B 115, 9739 (2011). - J.-S. McEwen, P. Gaspard, Y. De Decker, C. Barroo, T. Visart De
Bocarmé and N. Kruse
Catalytic reduction of NO2 with hydrogen on Pt field emitter tips: Kinetic instabilities on the nanoscale, Langmuir 26, 16381 (2010). - Y. De Decker and F. Baras
Bistability and explosive transients in surface reactions: The role of fluctuations and spatial correlations, EPJ B 78, 173 (2010). - X. Guo, Y. De Decker and J.W. Evans
Metastability in Schloegl's second model for autocatalysis: Lattice-gas realization with particle diffusion, Phys. Rev. E 82, 021121 (2010). - Y. De Decker, J.B. Benziger, E. Kimball and I.G. Kevrekidis
Multistability, nonlinear response and wave propagation in self-humidified PEM fuel cells, Chem. Eng. Sci. 65, 2399 (2010). - Th. Doneux and Y. De Decker
A simple model to describe the effect of electrostatic interactions on the composition of mixed self-assembled monolayers, Langmuir 25, 2199 (2009). - J.B. Benziger, E.-S. Chia, Y. De Decker and I.G. Kevrekidis
Ignition and front propagation in polymer electrolyte membrane fuel cells, J. Phys. Chem. C 111, 2330 (2007). - Y. De Decker and A.S. Mikhailov
Nanoscale pattern formation in non-equilibrium surface chemical reactions, Prog. Theoret. Phys. Supp. 165, 119 (2006). - Y. De Decker and A.S. Mikhailov
Promoter-induced nonlinear pattern formation in surface chemical reactions, J. Phys. Chem. B 108, 14759 (2004). - Y. De Decker, H. Marbach, M. Hinz, S. Günther, M. Kiskinova,
A.S. Mikhailov and R. Imbihl
Promoter-induced reactive phase separation in surface reactions, Phys. Rev. Lett. 92, 198305 (2004). - Y. De Decker, G.A. Tsekouras, A. Provata, Th. Emeux and G.
Nicolis
Propagating waves in one-dimensional discrete networks of coupled units, Phys. Rev. E 69, 036203 (2004). - Y. De Decker, F. Baras, N. Kruse and G. Nicolis
Modeling the NO+H2 reaction on a Pt field emitter tip: Mean-field analysis and Monte Carlo simulations, J. Chem. Phys. 117, 10244 (2002).
