 |
Professor Nonlinear Physical Chemistry Unit Service de Chimie Physique et Biologie Théorique Faculté des Sciences Campus Plaine, C.P. 231 Université libre de Bruxelles, 1050 Brussels, Belgium ☎ +32 (0)2 650 5750 📠 +32 (0)2 650 5767 ✉ fabian.brau@ulb.be |
Matter can be functionalized to meet specific needs by modifying its shape or structure. This task can be relatively simple such as, for example, when a piece of glass is cut into a lens. However, manipulating objects or structuring surfaces at nano- or microscale are complex tasks, sometimes expensive and which often require advanced equipment. Parts of my research aims to develop new methods based on the theory of pattern formation and self-organization/self-assembly for manipulating matter and creating complex structures. The recent interest in these methods is fueled, among other things, by the potential impact that efficient patterning methods could have on modern surface-based technologies, such as the fabrication of optical and microfluidic devices or components for deformable electronics. Indeed, using self-organized processes to create the desired structures spontaneously, without local actions on matter, reduces significantly the production costs and the fabrication time. Understanding the mechanisms governing the emergence of these forms and identifying the parameters controlling their shapes are the main tasks in order to create tailored (meta)materials. To achieve this objective, I use various strategies involving elastic thin films or the interaction between fluids and solids (fluid-structure interaction).
Nature can also be a source of inspiration because it offers many examples of complex shapes created spontaneously. However, in a natural system, the observed structures may be due to multiple causes acting together. To understand these complex forms, it is necessary to isolate fundamental morphologies produced by a single specific and controlled stress. My approach is therefore to design simple model systems capturing the essential physics to describe some characteristics of more complex systems. From a fundamental perspective, my research contributes to highlight common formation mechanisms of structures emerging in various systems (natural or technological). These studies help to unify our understanding of the origin of forms emerging spontaneously. This allows to describe a maximum of observations with minimum basic principles. These studies are therefore intrinsically interdisciplinary, since they offer analysis tools which can be used in fields ranging from biology to geophysics. From an applied perspective, understanding these formation mechanisms allows to control the emergence and the shape of these patterns to structure materials according to the needs in a simple, inexpensive and fast way.
Recently, parts of my research focus on the physics of capture of viscous fluids by small animals such as bees. Capturing viscous fluid at small scales is a challenge that nectarivores have solved by developing various type of specialized tongues or proboscis. These appendages typically consist of a complex assembly of flexible structures having a small size compared to the capillary length. Interesting elastocapillary problems appear thus in this context. Our strategy is to carry out systematic experimental and theoretical studies of elastocapillary effects coupled with viscous flows for a series of simple model systems mimicking biological systems.
Past members
| Lucie
Domino (Postdoc ARC 2022-2023) |
CNRS researcher at IUSTI laboratory (Aix-Marseille University) |
| Basile Radisson (Postdoc ARC 2022-2023) | Postdoc at LiPhy laboratory (Grenoble University) [E. Siéfert] |
| Hadrien Bense (Postdoc ARC + Marie Sklodowska-Curie fellowship 2022-2023) | CNRS researcher at LAUM laboratory (Le Mans University) |
| Giulio Facchini (Postdoc FNRS 2021-2023) | CNRS researcher at MSC laboratory (Paris University) |
| Emmanuel Siéfert (Postdoc ARC + Marie
Sklodowska-Curie fellowship + FNRS 2021-2023) |
CNRS researcher at LiPhy laboratory (Grenoble University) |
| Hoa-Ai
Béatrice Hua (PhD Student PDR 2019-2023) |
Postdoc at GRASP
laboratory (Liège University) [N. Vandewalle] |
| Alessandro Comolli (Postdoc Prodex
+ Cofund 2018-2022) |
Scientific officer at European Research Council |









Honey bees can collect nectar by dipping their hairy tongues into it, or they can attach their tongue to the wall of long corollas and directly suck the nectar along the tongue sides. The honey bee feeding apparatus is unveiled as a multifunctional tool that can switch between lapping and sucking nectar according to the instantaneous ingesting efficiency, which is determined by the interplay of nectar–mouth distance and sugar concentration.
PNAS 2023
Press
coverage: Science
Bees capture nectar by dipping their tongue into 5 times per second (see snapshots, \(\Delta t = 20\) ms). Among the 20000 bee species known to date, almost half have a long tongue of radius \(R_G\) decorated with slender papillae of length \(L\) and radius \(R\). Experiments performed under a microscope equipped with a high speed camera show that when the tongue is immersed in the nectar, the papillae open like the hairs of a brush. The presence of these outgrowths help to trap a larger quantity of nectar compared to a bare tongue. These same experiments show, however, that this morphological advantage no longer plays any role when the sugar content of the nectar exceeds a critical value. Indeed, when the sugar concentration increases, the viscosity of the nectar increases rapidly and prevents the papillae from fully opening before the tongue withdraws from the nectar (panel (a)). The motion of the papillae in a viscous liquid has been modeled using an approach based on fluid mechanics and the elasticity of rods. This theoretical model combined with measurements of the morphology of the tongue allow to explain why the ingestion rate of nectar in bees, \(Q\), drops sharply at sugar concentration above 50-60%. The theoretical predictions are confirmed by in vivo measurements of these ingestion rates carried out on several bee species (panel (b)). This study shows that the value \(c_s^*\) of the sugar concentration at which the absorption of calories is optimal is determined by the ratio \(L/R\) whereas the value \(c_s^m\) of the sugar concentration at which the ingestion rate is minimum is essentially universal and around 72% (panel (c)).
PNAS 2021

Reaction-diffusion (RD) fronts are ubiquitous in a wide variety of phenomena ranging from population dynamics and disease spreading to image processing and nanotechnology to name a few. Among the large family of RD fronts, \(A+B \to C\) fronts are observed when initially separated reactants \(A\) and \(B\) meet by diffusion and react to produce \(C\). Depending on the nature of \(A\) and \(B\), their dynamics is representative of many problems in chemistry, geochemistry, finance and many others. The temporal evolution of the front position, \(x_f\), defined as the location of maximum \(C\) production, of the front width \(w\) and of the local production rate, \(R(x_f)\), have long been derived theoretically and confirmed experimentally. The related scalings \(x_f \sim t^{1/2}\), \(w\sim t^{1/6}\) and \(R(x_f) \sim t^{-2/3}\), form the basis of \(A+B \to C\) RD front theory confirmed in many applications. In flows, \(A+B \to C\) processes provide another important class of dynamics, e.g. in combustion and environmental problems. The coupling between convection and reaction leads to complex dynamics when the flow, actively influenced by transported species, feedbacks on their spatio-temporal distribution. The radial advection of reacting species is currently receiving growing attention. For example, \(A+B \to C\)-type precipitation reactions in a radial flow give rise to a large variety of complex self-assembled structures, to thermodynamically unstable crystalline forms, or to microstructures significantly different from those obtained in homogeneous systems. Similarly, a suitable redefinition of distance may recast some transport phenomena into a radial spreading as done in studies of infectious disease spreading. Motivated by the broad applications of radial transport in reactive systems, the dynamics of \(A+B\to C\) fronts is analyzed theoretically in presence of passive advection when \(A\) is injected radially into \(B\) at a constant inlet flow rate \(Q\). We compute the long-time evolution of the front position, \(r_f\), of its width, \(w\), and of the local production rate \(R\) of the product \(C\) at \(r_f\). We show that, while advection does not change the well-known scaling exponents of the evolution of corresponding RD fronts, their dynamics is however significantly influenced by the injection. In particular, the total amount of product, \(n_{\text{C}}\), varies as \(n_{\text{C}} \sim Q^{-1/2}\, V\), where \(V\) is the volume of injected reactant. This control strategy paves the way to a flow control of the amount and spatial distribution of the reaction front product. It compares well with calcium carbonate precipitation experiments for which the amount of solid product generated in flow conditions at fixed concentrations of reactants can be tuned by varying the flow rate.
Physical Review Letters 2017
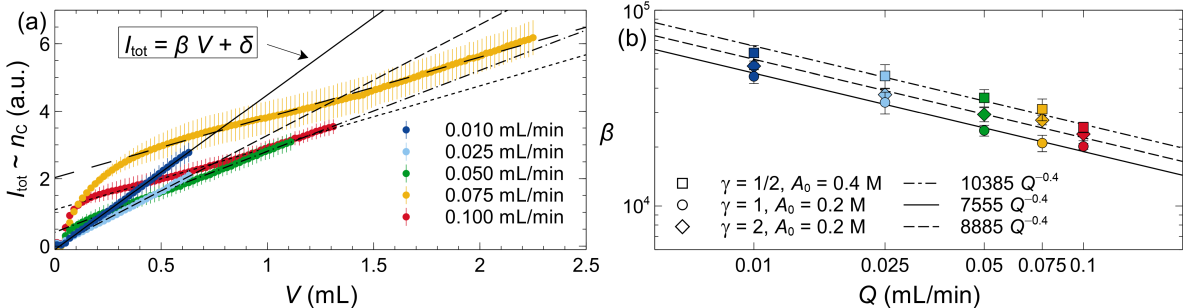 |
| Precipitation reaction by radial injection in a confined quasi-2D reactor of a solution of carbonate ions (\(A=\) CO\(_3^{2-}\)) into a solution of calcium ions (\(B=\) Ca\(^{2+}\)) producing calcium carbonate (\(C=\) CaCO\(_3\)). (a) \(n_{\text{C}}\), estimated by the intensity \(I_{\text{tot}}\) of light reflected by \(C\), as a function of the volume \(V\) of the injected Na\(_2\)CO\(_3\) solution for various \(Q\) and initial concentrations \(A_0=B_0=0.2\) M. (b) Slope \(\beta\) of the asymptotic linear regime shown in (a) as a function of \(Q\) for various \(\gamma = B_0/A_0\). |
Nature Physics 2016
 |
Press coverage: The
Washington Post — The
New-York Times — Los
Angeles Times — The
Times of India — New-Zealand
Herald — The
Japan Times — National
Geographic — Science
— Nature
— Le
point — Le
Monde — Le
Soir — Pour
La Science —
Science
& Avenir —
Scientific
American — Phys.org
—
DailyScience — ArsTechnica
— Nature
World News — Business
Insider — Wissenschaft
Aktuell — La
Province
Twisted ribbons. Twisted ribbons under tension exhibit a remarkably rich morphology, from smooth and wrinkled helicoids, to cylindrical or faceted patterns. This complexity emanates from the instability of the natural, helicoidal symmetry of the system, which generates both longitudinal and transverse stresses, thereby leading to buckling of the ribbon. Here we focus on the tessellation patterns made of triangular facets. Our experimental observations are described within an "asymptotic isometry'' approach that brings together geometry and elasticity. The geometry consists of parametrized families of surfaces, isometric to the undeformed ribbon in the singular limit of vanishing thickness and tensile load. The energy, whose minimization selects the favored structure among those families, is governed by the tensile work and bending cost of the pattern. This framework describes the coexistence lines in a morphological phase diagram, and determines the domain of existence of faceted structures.
Physical Review Letters 2016
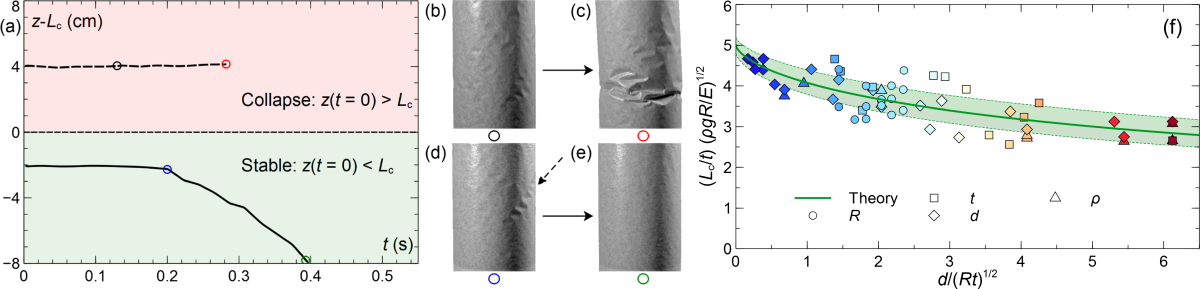
Structure stability under flows. Experiments were carried out to investigate the collapse of cylindrical shells of variable radius \(R\) and thickness \(t\) under the action of granular flows (size \(d\), density \(\rho\)). The critical heights, \(L_c\), of the granular material column beyond which the structure collapses when its content flows freely through a circular hole of given size at the base of the structure have been measured. It has been shown that the silos could withstand loads (height of the granular column) significantly higher than those obtained by an estimate based on standard theories of stability of shells and stress distribution in granular columns (Janssen model). Figure (a) shows the position of the upper layer of grains \(z\) as a function of time measured relatively to the collapse height, \(L_c\). When \(L > L_c\), irreversible deformations occur leading to a collapse of the silo. The circles indicate the time at which pictures (b)–(e) are taken. The pictures (b)–(c) show two states occurring during discharge onset for \(L > L_c\). Panel (c) shows a collapsed silo. The pictures (d)–(e) show two states occurring at the discharge onset and once the discharge is completed for \(L < L_c\). The solid arrows indicate the temporal evolution and the dashed arrow shows localized diamond dimples smoothed out during the discharge. It was also shown that the critical height decreases when the grain size \(d\) increased. The model describing the fluid-structure interaction taking into account the actual status of friction forces on the walls and deformation of the structure due to the finite size of the grains. This completely analytical model allowed to describe the experimental data of this complex problem satisfactorily, see figure (f) where each symbol shows the quantity which is varied.
Physical Review Letters 2015
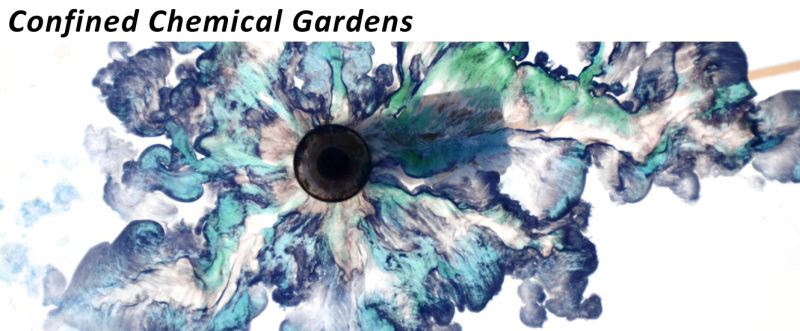
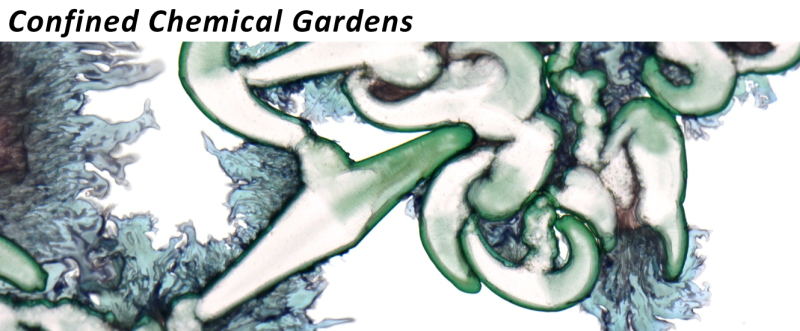
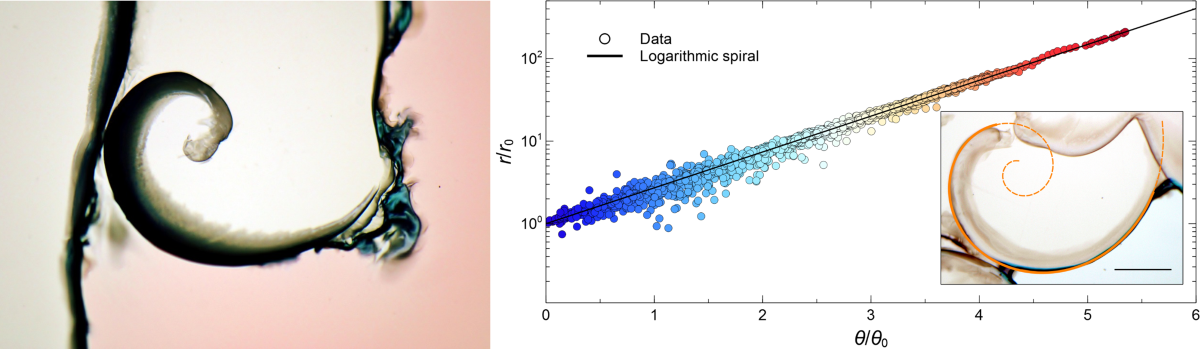
Confined chemical gardens: From chemistry to structures. Chemical gardens are formed by the spontaneous assembly of mineral precipitates generated when a metal salt is dissolved in an alkaline solution. Irregular and multicolored tubular structures rising then spontaneously by the combined action of different physical processes (osmotic pressure, gravity effects, reactions and diffusion) responsible for the irregularity and lack of reproducibility of the shapes obtained. In order to gain reproducible, we grew chemical gardens in a quasi two-dimensional confined by injecting a reagent into the other between two horizontal plates (Hele-Shaw cell). The experiments performed by F. Haudin showed that, in such conditions, a rich collection of reproducible structures can be obtained including, in particular, spiral structures. A geometric model has been developped to understand the formation of these spiral pattern. This model predicts that the spirals should be logarithmic whose polar equation is \(r = r_0 \exp(\theta/\theta_0)\). The figure below shows the evolution of radial distances \(r\), measured from the center of the spiral, as a function of the polar angle \(\theta\). The agreement between the theoretical prediction and experimental data is excellent (see figure below).
PNAS 2014
Press
coverage: Physics
Today — Le Monde — La
Recherche n°495 — Athena
— Phys.org
— DailyScience
— Chemistry
World
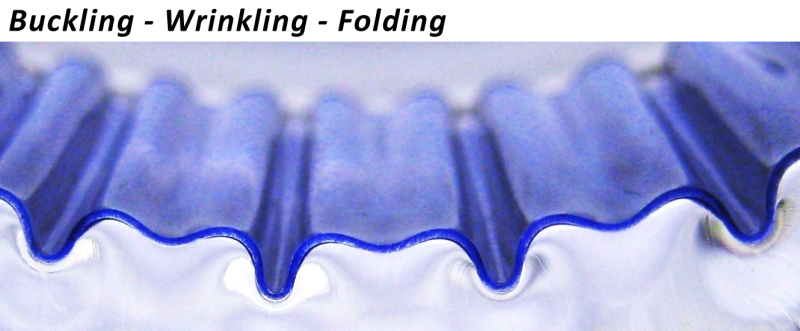
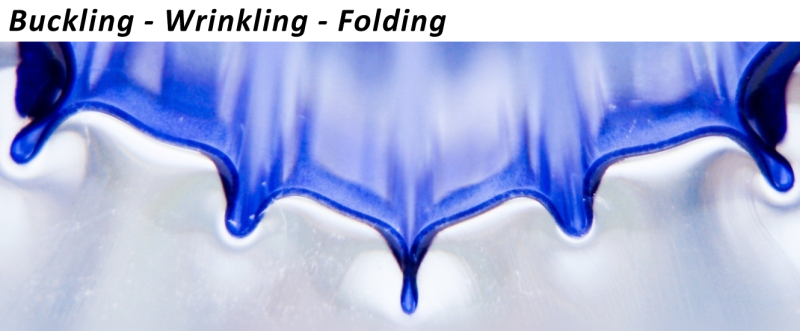
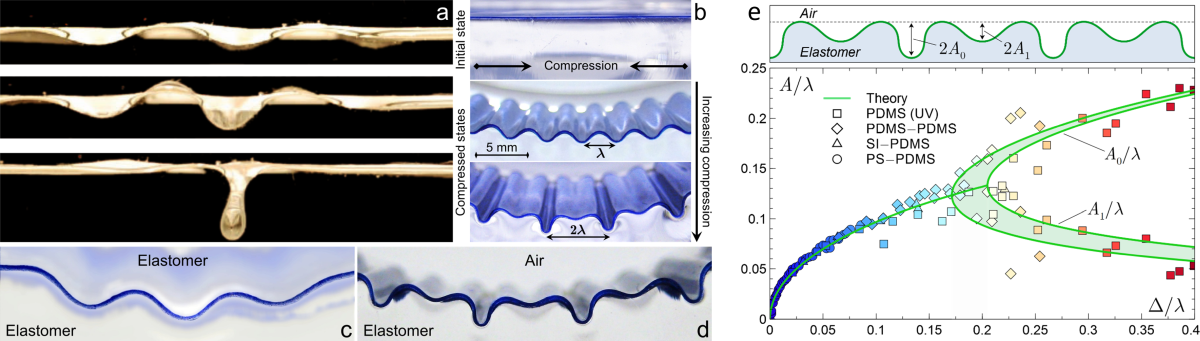
From fingerprints to mountain ranges. Systems involving a thin rigid sheet attached to a soft and thick substrate are ubiquitous in nature. When such a system is compressed in the plane of the sheet, wrinkles spontaneously appear and allow to explain, for example, the formation of wrinkles on the skin, mountain ranges and fingerprints. The wavelength of these undulations depends on the material properties: \(\lambda \sim h (E/K)^{1/3}\) for an elastic substrate and \(\lambda \sim h^{3/4} (E/\rho g)^{1/4}\) for a liquid substrate, where \(h\) is the sheet thickness, \(E\) is the sheet Young modulus, \(K\) and \(\rho g\) are the effective stiffness of the substrate. For an elastic substrate, if the system is further compressed, a totally new and unexpected phenomenon is observed. The folds formed are separated into two families: the amplitude of one fold increases to the detriment of its two neighbors (b,d). One fold every two folds concentrates the deformation energy to create a structure with a period twice the initial period. If the compression is increased, the same process is repeated to yield a quadrupling of the initial period. The up-down symmetry breaking induced by the elastic substrate is an essential ingredient to explain the period doubling. If the substrate is a fluid (a) or if the sheet is sandwiched between two identical soft foundations (c), the system does not break this symmetry and the period doubling instability is not observed. The experimental evolution of the pattern amplitudes \(A\) as a function of the compression ratio \(\delta = \Delta/L\), where \(\Delta\) is the horizontal displacement of the sheet and \(L\) its length, is shown in figure (e) and compares satisfactorily with the theoretical evolution.
Nature Physics 2011
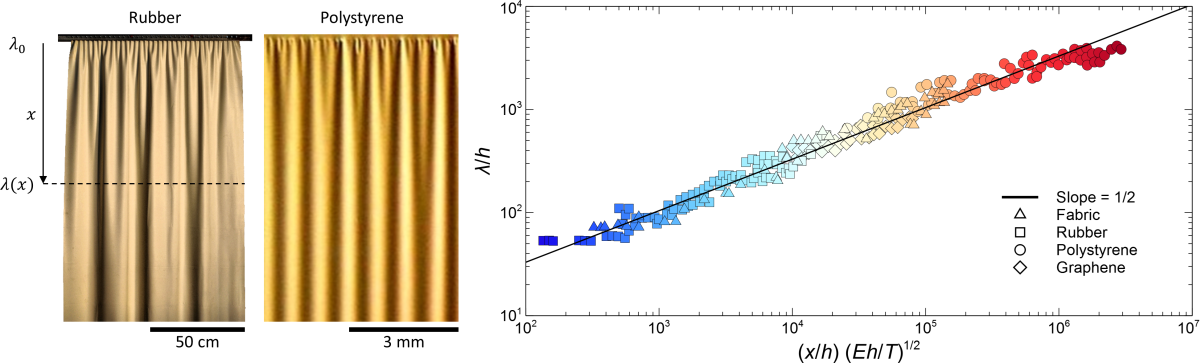
Hierarchy of folds: From graphene to curtains. Thin films are unstable when they are compressed. If a thin sheet of thickness \(h\) and Young modulus \(E\), is forced to adopt a periodic profile, with a wavelength \(\lambda_0\), along one of its edges, this constraint will be relaxed gradually as one moves away from this edge producing a cascade of folds of increasing size which progressively merge two by two. Regions where these folds merge were named "wrinklons" and represent the building blocks of the pattern. Using these wrinklons, we showed how to reconstruct the complete pattern and determine the evolution of the mean wavelength \(\lambda\) as a function of the distance to the constrained edge \(x\). When tension \(T\) in the sheet, which can be due to the weight of the sheet, is negligible then \(\lambda \sim x^{2/3}\) otherwise \(\lambda \sim x^{1/2}\). This wavelength evolution has been compared with experimental data spanning five orders of magnitude for the imposed wavelength \(\lambda_0\), the sheet thickness \(h\) or the system size (see figure below for "heavy" sheets).
Physical Review Letters 2011
Press
coverage: Le
Soir — Physics
Synopsis — Physics
World — Science
& Avenir n° 773 — Pour
La Science n°405
How geometry controls fractures in thin adhesive films. One can take off a piece of adhesive film attached to a substrate by making two parallel notches on one of its edges to creat a rectangular flap which is then pulled at a constant speed \(v\) and with a constant peeling angle \(\alpha\). When the substrate is flat, it has been shown that the initially parallel cracks converge toward each other along a linear path until they meet. The shape of the flap detached from the substrate is then invariably triangular (a). The angle \(2\theta\) formed by the two cracks at the meeting point depends on the material properties: \(\sin \theta \sim (E t \tau)^{1/2}/ \gamma\), where \(E\) is the Young modulus, \(t\) is the sheet thickness, \(\tau\) is the adhesive energy per unit area and \(\gamma\) is the work of fracture of the film. We have shown that when the substrate has a finite curvature \(\kappa=1/R\), the forms adopted by the flap are much more diverse and it is even possible to obtain diverging paths for the two fractures (b, c, d). This finite curvature induces a finite geodesic curvature along the debonding front \(\bar{\kappa} = 1/\bar{R} = \kappa \cot(\alpha/2)\) which vanishes when the peeling angle is equal to \(180^{\circ}\) (in that case, we recover the same shape for the flap than the one obtained for flat substrates). The agreement between the data and the developed geometrical model is remarkable (e, f).
Physical Review Letters 2011

Press
coverage: Le
Soir
 |
 |
|
 |
 |
- H. Bense, E. Siéfert, F. Brau
Measurement of capillary forces using two fibers dynamically withdrawn from a liquid: Evidence for an enhanced Cheerios effect, Phys. Rev. Lett. 131, 184003 (2023).
Editor's suggestion + Physics Magazine - J. Wei, A. Rico-Guevara, S. W. Nicolson, F. Brau, P. Damman,
S. N. Gorb, Z. Wu, J. Wu
Honey bees switch mechanisms to drink deep nectar efficiently, PNAS 120, e2305436120 (2023).
Press (see above)
- J. Cappello, B. Scheid, F. Brau, E. Siéfert
Bioinspired shape shifting of liquid-infused ribbed sheets, PNAS 120, e2216001120 (2023). - G. Kozyreff, E. Siéfert, B. Radisson, F. Brau
The θ-formulation of the two-dimensional elastica: buckling and boundary layer theory, Proc. R. Soc. A 479, 20230087 (2023). - G. Kozyreff, B. Davidovitch, S. G. Prasath, G. Palumbo, F.
Brau
Effect of external tension on the wetting of an elastic sheet, Phys. Rev. E 107, 035101 (2023). - E. Siéfert, H.-A. B. Hua, F. Brau
Capillary coalescence of two partially immersed slender structures, Extreme Mech. Lett. 55, 101823 (2022). - J. Wei, F. Brau, P. Damman, A. Draux, H.-A. B. Hua, Z. Wu, J.
Wu
Trade-off mechanism of honey bee sucking and lapping, Soft matter 18, 5568 (2022).
Cover - A. Draux, H.-A. B. Hua, P. Damman, F. Brau
Relaxation dynamics of a flexible rod in a fluid, Phys. Rev. Fluids 6, 114102 (2021). - A. Lechantre, A. Draux, H.-A. B. Hua, D. Michez, P. Damman, F.
Brau
Essential role of papillae flexibility in nectar capture by bees, PNAS 118, e2025513118 (2021).
Press (see above) - F. Brau & A. De Wit
Influence of rectilinear vs radial advection on the yield of A + B → C reaction fronts: A comparison, J. Chem. Phys. 152, 054716 (2020). - A. Comolli, A. De Wit & F. Brau
Dynamics of A + B → C reaction fronts under radial advection in three dimensions, Phys. Rev. E. 100, 052213 (2019). - F. Brau, S. Thouvenel-Romans, O. Steinbock, S. S. S. Cardoso
& J. H. E. Cartwright
Filiform corrosion as a pressure-driven delamination process, Soft Matter 15, 803 (2019). - P. M. Reis, F. Brau & P. Damman
The mechanics of slender structures, Nature Physics 14, 1150 (2018).
Comment - F. Brau, F. Haudin, S. Thouvenel-Romans, A. De Wit, O.
Steinbock, S. S. S. Cardoso & J. H. E. Cartwright
Filament dynamics in confined chemical gardens and in filiform corrosion, Phys. Chem. Chem. Phys. 20, 784 (2018).
2018 PCCP HOT Articles - F. Brau, G. Schuszter & A. De Wit
Flow control of A + B → C fronts by radial injection, Phys. Rev. Lett. 108, 134101 (2017). - G. Schuszter, F. Brau & A. De Wit
Flow-driven control of calcium carbonate precipitation patterns in a confined geometry, Phys. Chem. Chem. Phys. 18, 25592 (2016). - H. Pham Dinh, V. Démery, B. Davidovitch, F. Brau & P.
Damman
From cylindrical to stretching ridges and wrinkles in twisted ribbons, Phys. Rev. Lett. 117, 104301 (2016). - F. Brau, D. Lanterbecq, L.-N. Zghikh, V. Bels & P. Damman
Dynamics of the prey prehension by chameleons through viscous adhesion, Nature Physics 12, 931 (2016).
Press (see above) - G. Schuszter, F. Brau & A. De Wit
Calcium Carbonate Mineralization in a Confined Geometry, Environ. Sci. Technol. Lett. 3, 156 (2016). - G. Gutiérrez, C. Colonnello, P. Boltenhagen, J. R. Darias, R.
Peralta-Fabi, F. Brau & E. Clément
Silo collapse under granular discharge, Phys. Rev. Lett. 114, 018001 (2015). - O. Oshri, F. Brau & H. Diamant
Wrinkles and folds in a fluid-supported sheet of finite size, Phys. Rev. E 91, 052408 (2015).
Editors' Suggestion - F. Haudin, V. Brasiliense, J. H. E. Cartwright, F. Brau &
A. De Wit
Genericity of confined chemical garden patterns with regard to changes in the reactants, Phys. Chem. Chem. Phys. 17, 12804 (2015). - F. Haudin, J. H. E. Cartwright, F. Brau & A. De Wit
Spiral precipitation patterns in confined chemical gardens, PNAS 111, 17363 (2014).
See Commentary by Oliver Steinbock + Press (see above)
- F. Brau
Tearing of thin sheets: Cracks interacting through an elastic ridge, Phys. Rev. E 90, 062406 (2014). - F. Brau, P. Damman, H. Diamant & T. A. Witten
Wrinkle to fold transition: Influence of the substrate response, Soft Matter 9, 8177 (2013). - B. Kolaric, S. Desprez, F. Brau & P. Damman
Design of curved photonic crystal using swelling induced instabilities, J. Materials Chem. 22, 16205 (2012). - C. Zeng, F. Brau, B. Davidovitch & A. D. Dinsmore
Capillary interactions among spherical particles at curved liquid interfaces, Soft Matter 8, 8582 (2012). - A. Takei, F. Brau, J. Bico & B. Roman
Stretch-induced wrinkles in reinforced membranes: from out-of-plane to in-plane structures, EPL 96, 64001 (2011). - O. Kruglova, F. Brau, D. Villers & P. Damman
How geometry controls the tearing of adhesive thin films on curved surfaces, Phys. Rev. Lett. 107, 164303 (2011).
Editors' Suggestion - H. Vandeparre, M. Pineirua, F. Brau, B. Roman, J. Bico, C.
Gay, W. Bao, C. N. Lau, P. Reis & P. Damman
Wrinkling hierarchy in constrained thin sheets from suspended graphene to curtains, Phys. Rev. Lett. 106, 224301 (2011).
Editors' Suggestion + Cover + Press (see above) - F. Brau, H. Vandeparre, A. Sabbah, C. Poulard, A. Boudaoud
& P. Damman
Multiple-length-scale elastic instability mimics parametric resonance of nonlinear oscillators, Nature Physics 7, 56 (2011).
Press (see above) - U. Ebert, F. Brau, G. Derks, W. Hundsdorfer, C.-Y. Kao, C. Li,
A. Luque, B. Meulenbroek, S. Nijdam, V. Ratushnaya, L. Schäfer
& S. Tanveer
Multiple scales in streamer discharges, with an emphasis on moving boundary approximations, Nonlinearity 24, C1 (2011).
Cover - H. Vandeparre, S. Gabriele, F. Brau, C. Gay, K. K. Parker
& P. Damman
Hierarchical Wrinkling Patterns, Soft Matter 6, 5751 (2010). - F. Brau, B. Davidovitch & U. Ebert
Moving boundary approximation for curved streamer ionization fronts: Solvability analysis, Phys. Rev. E 78, 056212 (2008). - A. Luque, F. Brau & U. Ebert
Saffman-Taylor streamers: Mutual finger interaction in spark formation, Phys. Rev. E 78, 016206 (2008).
amplitude
boundary
concentration
confined
constant
crack
curvature
curved
deformation
displacement
elastic
energy
equation
evolution
experimental
field
flow
fluid
force
form
front
function
instability
interface
length
localized
membrane
model
nature
order
patterns
physical
precipitate
reactant
ridge
scale
shape
sheet
small
solution
spiral
structures
study
substrate
system
thin
transition
viscosity
wavelength
wrinkles
created at TagCrowd.com
